Information sheet
n°05b :
Formulae for the
calculation of the solar parallax
J.-E. Arlot, P. Rocher (IMCCE)
I.Introduction
In this
sheet, we will provide very simple formulae allowing to calculate an
approximate value of the solar parallax from two observations made from two
distant observing sites. Be careful that the choice of the observing site is
important. The information sheet n°05c provides explanations useful for the
choice of the two sites of observation
You will
find below two types of formulae :
Formulae
using the timing of two similar contacts between Venus and the Sun. Attention,
the two distant observations must concerned the same type of contact, either
the first external contacts, either the first internal contacts or the last
internal contacts or the last external contacts. This method requires a clock
in Universal Time in order to make the timings of the contacts in the same time
scale within one second of time. This method corresponds to the Delisle’s method.
Formulae using the duration between two similar contacts. With this method, we will take into account the duration of the transit of Venus in front of the Sun, measured between two similar contacts, either between the two external contacts (difficult), or between the two internal contacts. This method does not need to have the Universal Time scale available since only the duration is needed. However, this method requires that the two observing sites be able to observe the beginning and the end of the transit. This method corresponds to the Halley’s method.
Pre-calculated coefficients
In order to provide very simple formulae, we pre-calculated the parameters A, B et C which do not depend on the positions of the observers but which take into account the motions of Venus and the Earth around the Sun and the motion of the Earth around its axis. The parameter dD/dt represents the instantaneous variation of the distance from the center of Venus to the center of the Sun. Then, a calculation of triangulation is possible between the two observing sites, Venus and the Sun (even not in the same plane) and using the third Kepler’s law to determinate the relationship between the Eart-Sun distance and the Venus-Sun distance.
|
Description of the contact |
A |
B |
C |
dD/dt "/min |
|
First external contact (index 1) |
2,2606 |
-0,0194 |
1,0110 |
-3,0846 |
|
First internal contact (indice 2) |
2,1970 |
0,2237 |
1,1206 |
-2,9394 |
|
Last internal contact (index 3) |
-1,0929 |
-1,1376 |
1,9090 |
2,9391 |
|
Last external contact (index 4) |
-0,9799 |
-1,3390 |
1,8383 |
3,0842 |
Table n°1
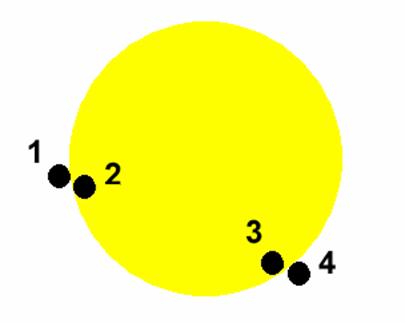
Successive contacts between Venus and the Sun
II Observation of the
contacts
You need the following
numerical data :
-
latitudes and longitudes of the two observing
sites (l1, j1; l2, j2)
-
the timings of the contacts « i »
(i=1, 2 , 3 or 4) observed at the sites 1 and 2 in the same time scale –referred
to Universal Time- (ti,1 ; ti,2)
The formula is as follows :
[Ai (cos j1 cos l1 – cos j2 cos l2) + Bi (cos j1 sin l1 – cos j2 sin l2)
+ Ci (sin j1 – sin j2)] p0 = – dD/dt (ti,1 – ti,2)
To make the calculation, follow
the calculation sheet “Delisle” below.
III Observation of the
durations
You need the following
numerical data :
-
latitudes and longitudes of the two observing
sites (l1, j1; l2, j2)
-
the difference DT of the observed durations of the
transit from the two observing sites (duration from site 1 – duration from site
2) corresponding to the contacts « i and j » (i,j=1,4 for the
external contacts or 2,3 for the internal contacts) observed from the sites 1
and 2
-
The formula is as follows :
[(Ai+Aj) (cos j1 cos l1 – cos j2 cos l2)
+ (Bi+Bj) (cos j1 sin l1 – cos j2 sin l2)
+ (Ci+Cj) (sin j1 – sin j2)] . p0
= – DT . dD/dt
To make the calculation,
follow the calculation sheet “Halley” below.
Calculation sheet « Delisle » :
Calculation of the solar
parallax using the observation of the contacts from two observing sites
You need the following
numerical data :
-
latitudes and longitudes of the two observing
sites (l1, j1; l2, j2)
-
the timings of the contacts « i »
(i=1, 2 , 3 ou 4) observed at the sites 1 and 2 in the same time scale –referred
to Universal Time- (ti,1 ; ti,2)
Formula (F1) is as follows :
[Ai (cos j1 cos l1 – cos j2 cos l2)
+ Bi (cos j1 sin l1 – cos j2 sin l2)
+ Ci (sin j1 – sin j2)] p0
=
– dD/dt (ti,1 – ti,2)
To make the
calculation,please fill up the calculation sheet “Delisle” below. Attention, one
sheet for two observations of the same contact « i »
Longitude of the observing site n°1 : l1=
(1) Calculate the cosine of l1 : cos (l1) =
(2) Calculate the sine of l1 : sin (l1) =
Latitude of the observing site n°1 : j1 =
(3) Calculate the cosine of j1 : cos (j1) =
(4) Calculate the sine of j1 : sin (j1) =
Longitude of the observing site n°2 : l2=
(5) Calculate the cosine of l2 : cos (l2) =
(6) Calculate the sine of l2 : sin (l2) =
Latitude of the observing site n°2 : j2 =
(7) Calculate the cosine of j2 : cos (j2) =
(8) Calculate the sine of j2 : sin (j2) =
(9) Calculate (cos j1 cos l1) = line (3) x line
(1) =
(10) Calculate (cos j2 cos l2) = line (7) x line
(5) =
(11) Calculate (cos j1 cos l1 – cos j2 cos l2) = line (9) – line
(10) =
(12) Calculate (cos j1 sin l1) = line (3) x line
(2) =
(13) Calculate (cos j2 sin l2) = line (7) x line
(6) =
(14) Calculate (cos j1 sin l1 – cos j2 sin l2) = line (12) – line
(13) =
(15) Calculate (sin j1 - sin j2 ) = line (4) – line
(8) =
Value of the index i for this calculation =
(16) Determinate Ai from table n°1 =
(17) Determinate Bi from table n°1 =
(18) Determinate Ci from table n°1 =
Now, determination of the numerical value of the first member of equation
(F1) :
(19) Calculate Ai x line (11) =
(20) Calculate Bi x line (14) =
(21) Calculate Ci x line (15)
=
(22) Calculate the first member of (F1) :
line (19) + line
(20) + line (21) =
(23) Observed timing of the contact « i » from site n°1 :
ti,1 =
(24) Observed timing of the contact « i » from site n°2 :
ti,2 =
(25) Difference between those two timings : (ti,1 –
ti,2) =
(26) Convert this difference into minutes anf fraction of minute =
(27) Determinate dD/dt from table
n°1 : dD/dt =
(28) Calculate the second member of equation (F1) :
line (27) x line (26) x (-1) =
(29) Calculate p0 = line (28) / line
(22) =
(30) Radius of the Earth = 6 378,1363 km
(31) Calculate now the astronomical unit :
AU = line (30) x 206265,806247
/ line (29) =
You may make the calculation
for each contact.
Calculation sheet
« Halley » :
Calculation of the solar parallax from the observation
of the duration of the transit from two different observing sites
You need the following
numerical data :
-
latitudes and longitudes of the two observing
sites (l1, j1; l2, j2)
-
the duration DT of the transit of Venus for each
of the two sites 1 and 2 corresponding to external contacts (i=1, j=4) or
internal (i=2, j=3)
-
the difference between the durations DT observed
from the two observing sites (duration site 1 – duration site 2) corresponding
to the external contacts (i=1, j=4) or internal (i=2, j=3)
Formula (F2) is as follows :
[(Ai+Aj) (cos j1 cos l1 – cos j2 cos l2)
+ (Bi+Bj) (cos j1 sin l1 – cos j2 sin l2)
+ (Ci+Cj) (sin j1 – sin j2)] . p0
= – DT . dD/dt
Longitude of the observing site n°1 : l1=
(1) Calculate the cosine of l1 : cos (l1) =
(2) Calculate the sine of l1 : sin (l1) =
Latitude of the observing site n°1 : j1 =
(3) Calculate the cosine of j1 : cos (j1) =
(4) Calculate the sine of j1 : sin (j1) =
Longitude of the observing site n°2 : l2=
(5) Calculate the cosine of l2 : cos (l2) =
(6) Calculate the sine of l2 : sin (l2) =
Latitude of the observing site n°2 : j2 =
(7) Calculate the cosine of j2 : cos (j2) =
(8) Calculate the sine of j2 : sin (j2) =
(9) Calculate (cos j1 cos l1) = line (3) x line
(1) =
(10) Calculate (cos j2 cos l2) = line (7) x line
(5) =
(11) Calculate (cos j1 cos l1 – cos j2 cos l2) = line (9) – line
(10) =
(12) Calculate (cos j1 sin l1) = line (3) x line
(2) =
(13) Calculate (cos j2 sin l2) = line (7) x line
(6) =
(14) Calculate (cos j1 sin l1 – cos j2 sin l2) = line (12) – line
(13) =
(15) Calculate (sin j1 - sin j2 ) = line (4) – line
(8) =
Value of the index i and j for the calculation : 1 and 4 for a
duration calculated from the external contacts ; 2 and 3 from the internal
contacts)
i =
j =
(16) Determinate Ai from table n°1 =
(17) Determinate Aj from table n°1 =
(18) Calculate Ai + Aj =
(19) Determinate Bi from table n°1 =
(20) Determinate Bj from table n°1 =
(21) Calculate Bi + Bj =
(22) Determinate Ci from table n°1 =
(23) Determinate Cj from table n°1 =
(24) Calculate Ci + Cj =
Now, determination of the numerical value of the first member of
equation (F1) :
(25) Calculate (Ai+Aj) x line (11) =
(26) Calculate (Bi+Bj) x line (14) =
(27) Calculate (Ci+Cj) x line
(15) =
(28) Calculate the first member of (F2) :
line (25) + line
(26) + line (27) =
(29) Duration of the transit observed from site n°1 =
(30) Convert this duration into minutes anf fraction of minute =
(31) Duration of the transit observed from site n°2 =
(32) Convert this duration into minutes anf fraction of minute =
(33) Difference between the durations : DT = line (30) – line (32)
=
(34) Determinate dD/dt from table
n°1 : dD/dt =
(by averaging the absolute values of the beginning i and the end j)
(35) Calculate the second member of equation (F2) :
line
(33) x line (34) x (-1) =
(choose the sign in order to have line (36) positive)
(36) Calculate p0 = line (35) / line
(28) =
(37) Radius of the Earth = 6 378,1363 km
(38) Calculate the astronomical unit :
AU = line (37) x 206265,806247
/ line (36) =
Let us
remind you that these methods are not completely accurate and that one should
use more complicated formulae for a better reduction of the data (cf. sheets
n°4 and 5). Even if your observations
are perfect, you will get only an approximate value of the astronomical unit. The
error may reach 10 to 20 millions kilometers if the observing sites are badly
chosen (proividing too close observed values for example –cf. sheet n°6-).